What is heat capacity?
Heat capacity (Specific) of gases is defined as the amount of heat required to raise the temperature of one gram of gases by unit degree but per mole of gas is called molar heat capacity or simply heat capacity. Usually, the heat capacity equation expressed at constant pressure (Cp) and volume (Cv) and energy unit is used for its calculation in physics or chemistry.
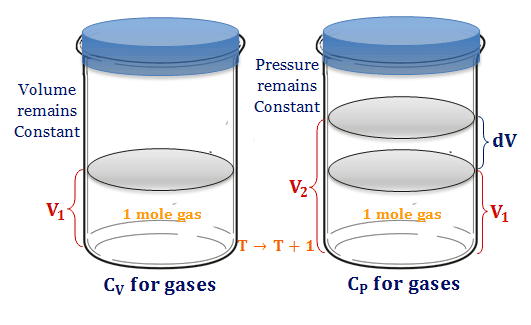
Monoatomic noble gas molecules like helium, neon, argon when heated in constant volume, the heat supplied will be utilized in increasing the translational kinetic energy because these molecules have no vibrational or rotational moment. These mono-atomic gases at the constant volume, no energy can be used to do any mechanical work. But if we heated in constant pressure the gas expands against the piston and does mechanical work. For polyatomic gases, the supplied heat uses not only translational kinetic energy but also vibrational or rotational energies.
Solids also have heat capacities measured from Dulong petit experimental data that the atomic heat of all crystalline solid elements is the constant quantity and approximately equal to 6.4 calories. Atomic heat is the product of specific heat and the atomic weight of the element. This law holds for many periodic table elements like silver, gold, aluminum, lead, iron, etc.
Heat capacity units
Specific heat capacity is an extensive property with unit J K-1 kg-1 because the amount of heat required to raise temperature depends on the mass of the substances. But molar heat capacity is an intensive property in thermodynamics having the unit J K-1 mol-1. We also use CGS and calories units to specify the heat capacities of the solid and gaseous substances. But if we maintained molar and specific heat capacity then per mole and per gram or kg used in these units.
Heat capacity at constant pressure
The amount of heat or thermal energy required to raise the temperature of one gram of a substance by 1°K is called specific heat and for one mole is called molar heat capacity. Therefore, Cp = M × cp, where Cp is measured at constant pressure and cp is their specific heat. From this formula, the temperature of one gm-mole of gas raised by one degree at constant pressure is called heat capacity at constant pressure or simply Cp.
Heat capacity at constant volume
Again from the definition, Cv = M × cv, where Cv is measured at constant volume, cv is their specific heat. Therefore, the temperature of one gm-mole of gas raised by one degree at constant volume is called heat capacity at constant volume or simply Cv.
Heat capacity in thermodynamics
Hence like internal energy, enthalpy, entropy, and free energy heat capacity also thermodynamic properties. Let dq energy required to increase the temperature dT for one mole of the gaseous substances. Therefore, the thermodynamic definition of the specific heat capacity, C = dq/dT, where dq = path function. Hence the values of heat change depend on the actual process which followed for this measurement. But we can place certain restrictions to obtain precise values of Cp and Cv. The usual restrictions are at constant pressure and at constant volume.
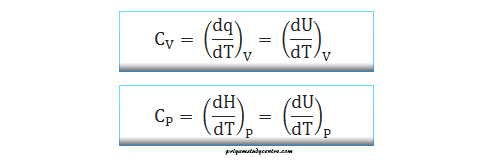
Cp and Cv values for gases
The calculation of Cp or Cv depends on the pressure and volume, especially in the cases of the properties of gases. Therefore, the observed quantity in the two operations would be different. Hence for measuring heat capacity, the condition of pressure and volume must have to specify. In learning chemistry and physics, Cp, Cv, and Cp/Cv or γ of some gases at 1 atm pressure and 298 K temperature are given below the table,
| Gases | Cp | Cv | γ |
| Argon (Ar) | 4.97 | 2.98 | 1.66 |
| Helium (He) | 4.97 | 2.98 | 1.66 |
| Mercury (Hg) | 5.00 | 3.00 | 1.67 |
| Hydrogen (H2) | 6.85 | 4.86 | 1.40 |
| Nitrogen (N2) | 6.96 | 4.97 | 1.40 |
| Oxygen (O2) | 7.03 | 5.03 | 1.40 |
| Carbon dioxide (CO2) | 8.83 | 6.80 | 1.30 |
| Sulfur dioxide (SO2) | 9.65 | 7.50 | 1.29 |
| water (H2O) | 8.67 | 6.47 | 1.34 |
| Methane (CH4) | 8.50 | 6.50 | 1.31 |
Problem: The Cp and Cv of gases are 0.125 and 0.075 cal gm-1 K-1 respectively, how to calculate the molecular weight and the gas formula from the specific heat equation. Name the gas if possible.
Solution: M = 40 and â‹Ž = 1.66(mono-atomic), Ar(Argon).
Mechanical work formula
A monoatomic gas can be heated at constant pressure and constant volume in a cylinder fitted with a piston. When the gas expands against the piston gives mechanical energy. In order to attain a 1° rise in temperature, the heat supplied should be sufficient to increase the energy of the molecules and also be able to do extra mechanical work.
Therefore, Cp equal to some mechanical energy required for lifting the piston from volume V1 to V2. CP – CV = mechanical work or energy = PdV = P(V2 – V1) = PV2 – PV1. If the gases obey ideal gas law, PV = RT. Therefore, Cp – Cv = R(T + 1) – RT, or Cp – Cv = R = 2 calories.
Heat capacity formula
Consider the monoatomic gases like argon or helium. If such gases are heated at constant volume, it is utilized for increasing the kinetic energy of the translation. Since the monoatomic gas molecules can not any absorption in vibrational or rotational motion. If no heat is used to do any mechanical work of expansion when the volume of the gas remains constant. Therefore, the Kinetic energy for one-mole ideal gases at T temperature, E = 3PV/2 = 3RT/2. Increase of kinetic energy for 1° rise in temperature for monoatomic gas helium or argon, ΔE = 3{R(T+1) – RT}/2 = 3R/2 =3 calories.
The heat supplied at a constant volume is equal to the rise in kinetic energy per unit degree rise in temperature. Therefore, Cv = ΔE = 3 calories. For one mole of monoatomic gas, the ratio of Cp/Cv universally expressed by the symbol γ calculated by the following equation, γ = Cp/Cv and Cp – Cv = R. Therefore, γ = (Cv + R)/Cv
= (3 + 2)/3 = 1.66.
Cp and Cv for polyatomic gas
For polyatomic molecules, the heat supplied is used up not only in increasing kinetic energy but also in increasing vibrational or rotational energies. Let x calories be used for increasing vibrational or rotational purposes.
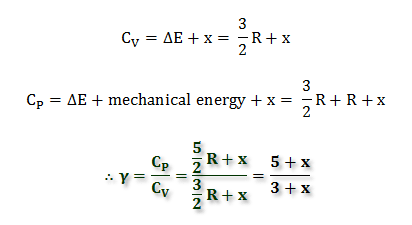
Cp – Cv = 2 calories remain constant for this energy equation but Cp/Cv calculation differing gas to gas.
Energy equation and specific heat capacity
Heat supplied to one gram-mole of a gas kept at a constant volume to increase the temperature by one degree has the Cv for monoatomic or polyatomic gases. But monoatomic gases use this energy to increase the translational kinetic energy and polyatomic gases use it to increases translational, vibrational, and rotational kinetic energy.
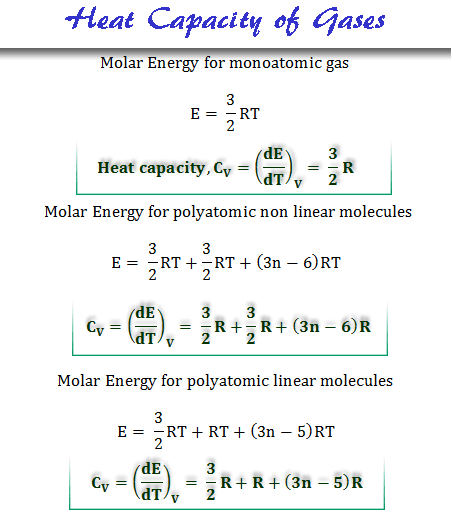
Heat capacity calculation
Experimental and calculation values of Cp and Cv revels due to the following facts. Due to the perfect arrangement of the monoatomic gases, Cv/R = 1.5. Therefore, the value of Cp and Cv independent of temperature over a wide range. For polyatomic gases, two points of disarrangement were found, the first always lower than the predicted value and the second is noticeable dependent on temperature. The observed values of heat capacity for polyatomic gases lie between the range of 2.5 to 3.5.
Classical mechanics does not describe the variation of these molecular properties. Therefore, we use quantum mechanics. The principle of equipartition is derived from the classical consideration of continuous energy absorption by atom governed by Maxwell distribution. Vibrational and rotational energy take place in discrete units, but the measured value of specific heat capacity of gas or gases is explained only on the basis of the quantum equation. At high temperatures, the energy levels are quite close and the observed spectrum would be continuous and the heat capacity equation for gas molecules would be valid.
